1. Physics and Philosophy
2. Ontological Problem of Quantum Mechanics
3. Mathematical Formalism of Quantum Mechanics
4. Wave-Particle Duality
5. Composition and Completeness of the Wavefunction
6. Superpositions of Eigenstates
7. Quantum Probability—Subjective or Objective?
8. Choice of Measurement Paradoxes
9. Heisenberg’s Uncertainty Principle
10. Particle Trajectories and Barrier Penetration
11. Energy-Time Uncertainty Relation
12. Physical Reality of Continuous Eigenstates
13. Metaphysical Wavefunctions and Virtual Particles
14. Young’s Double-Slit Experiment Reinterpreted
15. Sorting Out the Quantum Muddle
Quantum mechanics, to put it gently, is not the most philosophically lucid theory in physics. Its conventional interpretations include fantastic claims that strike at the realist and empiricist underpinnings of modern science. Among these claims are the principle of superposition,
where an object can be in an existentially indeterminate physical state, or simultaneously in contrary physical states; wave-particle duality,
where fundamental particles are thought to become wave-like in between observations; and a more general observation problem,
where the mere act of observing a quantum system necessarily alters it. These are just a few of the bizarre paradoxes of quantum mechanics. More troubling than their presence, perhaps, is the fact that most physicists do not perceive these paradoxes as problematic. If quantum mechanics contradicts Aristotelian logic and philosophical realism, so much the worse for logic and philosophy. Quantum mechanics has been empirically verified, we are told, so we must boldly embrace what is counterintuitive.
I maintain that these conventional interpretations of quantum mechanics—usually referred to as the Copenhagen interpretation
—are not merely counterintuitive, but also illogical, metaphysically incoherent, and unsupported by empirical evidence. This essay will show how conventional interpretations of quantum mechanics are not consistent or coherent in their existential treatment of fundamental particles, their wavefunctions, and physical states. Their non-binary logic is a gratuitous supposition that cannot be grounded in the mathematical basis of the theory, which is nothing more than linear algebra with Hermitian operators. The conflation of the wavefunction with palpably real particles blurs the line between mathematics and what is experimentally observed. This confusion has given rise to a more pernicious error of supposing that the deepest physical reality is wildly different in quality from what we actually observe, a thesis that strikes at the heart of scientific empiricism.
Concerns such as these are often dismissed by physicists, either because they are mere philosophy
and therefore have no place in physics, or because theoreticians are confident that the experimental validation of a mathematical theory gives them license to make any bizarre metaphysical assertion consistent with that theory. In contrast with the dry realism of the nineteenth century, theoretical physics in the last seventy years has become almost synonymous with impossible flights of fancy. The more illogical or counterintuitive a theory sounds, the more profound it is supposed to be. The proof, supposedly, is in the mathematical predictions and their confirmation. This attitude fails to recognize that a mathematical model admits of diverse metaphysical and physical interpretations.
Physics was not always opposed to philosophy; in fact, for most of its history it was part of philosophy, the part that studied nature (phusis). This marriage had the advantage of imposing conceptual coherence and logical rigor on the study of nature, but in the late classical and medieval periods it had the stifling effect of reducing physics to a merely formal analysis of abstract concepts (e.g., substantial forms, essences), with only tenuous ties to the palpable reality it was supposed to describe.
This essentialist philosophical approach to physics was challenged in the seventeenth century by Galileo, Gassendi, Descartes, and Newton, who all sought to explain natural mechanics without reference to any qualitative metaphysical system. Physical principles were instead to be expressed by mathematical relations among empirically observed and measured properties. The superiority of this new science appeared to be proved by the failure of traditional natural philosophy to account for Galileo’s astronomical observations, and by the fantastic predictive power of Newtonian mechanics, which reduced kinematic phenomena to a few elegant formulae. The fruitfulness of scientific investigations during the Age of Reason appeared to vindicate the emancipation of physics from philosophy.
Still, the separation of physics from philosophy was not a strict scientific necessity, but a cultural choice. Despite their professed aversion to philosophizing, modern physicists cannot avoid having metaphysical assumptions underlying their theories. The only difference is that now these assumptions are barely discussed by physicists, much less combined into a philosophically rigorous system. Such willful neglect of philosophy has not impeded the practical development of modern physics, insofar as it depends only on commonly accepted mathematical principles and replicable empirical data. This era of blissful philosophical na´vetÚ may no longer be sustainable, however, as modern physics approaches the limits of what can be physically observed, and directly engages metaphysical questions. Such is eminently the case with quantum mechanics, which deals with questions of ontology, the science of being.
What is really going on in nature when we are not looking? To suppose that such a question has a definite answer entails a belief in objective reality, that is, a reality outside of the thinking, perceiving subject. If we ask in what sense anything may exist or be when we are not looking, we are making an ontological inquiry, for we are not considering the attributes of this or that entity, but of the act or reality of being as such. When we ask broad questions about the reality of wavefunctions, which characterize every type of physical entity, we are really asking questions of ontology.
The most widely taught approach to interpreting quantum mechanics is the so-called Copenhagen interpretation,
which is actually a collection of varying, even contradictory, opinions proffered by Niels Bohr and Werner Heisenberg, among others. Common features of this interpretive approach include: (1) the wavefunction as a probabilistic description of phenomena; (2) Bohr’s complementarity principle,
where matter exists simultaneously and contradictorily as a wave and a particle; (3) the impossibility of knowing non-commuting properties at the same time, per the Heisenberg uncertainty principle; (4) the principle of superposition, where matter may exist simultaneously in two contrary well-defined states; (5) the collapse of the wavefunction
and various paradoxes where reality is altered by the act of observation.
Note that there is a great amount of tension among these principles. First and foremost, there is inconsistency about the reality of the wavefunction. If it is a measure of purely subjective probability, it makes no sense to appeal to it as physically real, as principles 2, 4, and 5 seem to do. If it measures an objective
probability (assuming such a concept can be made cogent), then it makes little sense to invoke the role of the subjective observer as altering reality, since we are not dealing with subjective probabilities. Second, if superposition
states are treated as objective realities per principle 4, then there is no reason to insist that matter is always particle-like, as the complementarity principle seems to imply. If the wavefunction is not a physical object, there is little reason to insist per the complementarity principle that matter is always wave-like. There are additional internal tensions beneath the surface, which can be seen only be examining the physico-mathematical theory in some detail, as we shall do later.
The Copenhagen interpretation is a muddled, barely coherent, quasi-subjectivist morass of metaphysical propositions. It simultaneously pretends to have nothing to do with philosophy and posits an ontological theory, a poor one at that. We can hardly expect better when an ad hoc philosophy is constructed by physicists without reference to any technically rigorous metaphysics. Bohr sought to preserve the theoretical independence of physics from philosophy by denying that we can know anything beyond experimental results, but this came at the expense of reducing physical theories and concepts to useful fictions for describing the behavior of real entities. Ultimately, all we definitely know from quantum mechanics is that its mathematics predicts the statistical outcomes of experiments. It teaches us nothing certain about what is really happening at the subatomic level in between measurements, or even if such a question has a definite answer.
Many physicists, in their disdain for philosophical constraints, try to have things both ways. Pointed philosophical questions about wave-particle duality or the objective reality of unobserved events are routinely dismissed as meaningless, unverifiable speculation. Yet in the next breath, a physicist may interpret the mathematical formalism of quantum mechanics to provide dubious answers to these supposedly meaningless questions. Emancipation from metaphysics has allowed physicists to assert logically incoherent theories as though they were profound, oscillating inconsistently between subjectivist and realist interpretations. Some have even claimed that quantum mechanics disproves the principle of non-contradiction! If abstract philosophical logic contradicts quantum mechanics, this only proves the limitations of standard logic, in the eyes of those convinced of the explanatory completeness of this physico-mathematical theory.
The inability or unwillingness of many physicists to see a viable alternative to the Copenhagen style of interpretation results from lack of exposure to any technically sophisticated metaphysics of being. Most scientists, like people in general, hold a commonsensical binary notion of existence and a mechanistic notion of causality, both of which break down under quantum mechanics. A technically sound philosopher, however, is aware of multiple modes of being (e.g., potency and act, possibility and necessity, esse and essentia), which may resolve quantum paradoxes without logical absurdity. Heisenberg, to his credit, attempted to explain quantum mechanical states as neo-Aristotelian potentia, but this aspect of his opinions never became part of the accepted consensus.[1] The philosopher Karl Popper adopted a vaguely similar theory of propensity,
based on his discussions with Einstein, but the cultural disconnect between philosophy and physics prevented his work from having much impact on the latter.
As a rule, physicists will listen only to other physicists about physics, so the only alternative ontological interpretations that have enjoyed some limited success in recent years have come from physicists.[2] A notably distinct interpretation was that of David Bohm (1917-92), who argued that the wavefunction is physically real. A more recent fashionable theory considers the time-reversed advanced wavefunction
as ontologically co-equal with the standard retarded wavefunction.
These alternative explanations have many merits, yet their vision is limited by the philosophical insularity of the physicists who formulated them. To reopen the dialogue between philosophy and physics, we must lay bare the underlying absurdities and misconceptions of Copenhagen-style theorizing, and then show how philosophically cogent thinking may provide a remedy.
As mentioned, the so-called Copenhagen interpretation of quantum mechanics encompasses a broad range of opinions. Some physicists believe that quantum paradoxes merely express the practical limitations of a measurement apparatus, while others see them as descriptions of fundamental physical reality. This diversity of opinion makes the Copenhagen interpretation ill-defined, resulting in conceptual confusion in the teaching of quantum mechanics. In practice, physics students interpret the theory however they or their professors see fit, but their real confidence is in the mathematics. The mathematical formalism of quantum mechanics, unlike its philosophical interpretations, has been experimentally verified as an excellent device for predicting probability distributions of events. As we shall see, several of the conventional interpretations of quantum mechanics are actually at odds with this mathematical theory and the logic upon which it rests.
Quantum mechanics, first and foremost, is a mathematicized theory of physics. The mathematics is not especially arcane or counterintuitive, for it is just the linear algebra of operators with complex-valued matrix elements. Linear algebra rests upon familiar principles of mathematical logic, including the principles of non-contradiction and the excluded middle. It would therefore be absurd to propose that quantum mechanical theory somehow transcends normal bivalent Aristotelian logic, when its mathematical structure in fact presupposes the validity of such logic.
In the mathematical formalism of quantum mechanics, physical states may be described as vectors in a complex Hilbert space. These state vectors are denoted using the shorthand ket
notation, where the state ψ is written as: |ψ⟩. It may also be represented more explicitly as a column matrix or vector. The Hermitian conjugate, a row matrix/vector, is denoted by a bra,
or ⟨ψ|. The kets
may be thought of as existing in a column vector space, and the bras
in a complementary row vector space. The norm of ψ is simply the product: ⟨ψ|ψ⟩1/2. We introduced the bras
in order to define the norm.[3] This is the extent of the purpose and meaning of the so-called dual space
of bras and kets; it has nothing to do with wave-particle duality, Schrödinger’s cat paradox, or any of the other purported dualisms
supposedly proven by quantum mechanics. The bra is simply the Hermitian mirror image of the ket. It makes no difference whether the bras or the kets are chosen to denote the wavefunction ψ, since the only physically observable quantities are the probabilities, which are found by squaring the magnitude of the components of ψ.[4] This operation is invariant under Hermitian conjugation, so the same result is achieved whether we choose to use the bras or the kets as representing physical states.[5] By convention, physicists choose the kets (or column vectors) to denote the state of a quantum system.
When we say that a complex-valued column vector or ket represents the state
or wavefunction,
we make some interesting assumptions. By treating |ψ⟩ as a state,
we attribute physical reality to a generally unobservable condition, as should be obvious from the fact that the coefficients of ψ may have imaginary values. The second name, ‘wavefunction,’ reflects the fact that ψ obeys the time-dependent Schrödinger equation, a simple second-order partial differential equation that has the same mathematical form as the classical wave equation. This is where we derive the notion of particles having a wave nature.
Properly understood, the wave-particle duality
is a duality between the wavefunction and the particle it describes. We should note, however, that the quantum wavefunction is not a physical wave! In the classical wave equation, ψ denotes a physically observable quantity, namely the amplitude of the wave. This may have spatial dimension, as in a mechanical wave, or dimensions of force per unit charge, as in electromagnetism. In quantum mechanics, ψ is a dimensionless, complex-valued mathematical object whose sole relation to physical reality is its squared magnitude, which equals the probability of occupying a particular state. Similarity in mathematical form does not imply similarity in physical interpretation.
The distinction between mathematical form and physics was made evident, for example, when the Michelson-Morley experiment forced physicists to abandon their belief in ether,
which arose in the first place from the conviction that light, being mathematically wavelike, must have a medium, as mechanical waves do. This analogy naturally fails, since the wave
amplitude of a photon does not extend in real space, but is a function of the strength of its electric and magnetic fields. Since the electromagnetic wave is not a perturbation of physical space, there is no reason to expect its conjectured medium
to exist there either.
The difference between a quantum wavefunction and a physical wave is perhaps less obvious when we consider the case where ψ is the spatial wavefunction, which measures the probability of being at position x, a continuous real variable. In several celebrated experiments, such as Young’s double-slit apparatus, the spatial distribution of scattered particle intensities resembles that of classical wave interference. Here, many physicists claim, is experimental evidence that the particle can become wavelike. Others, a bit more accurately, will say that the wavefunctions interfere with one another, while the particle remains a discrete object. I will deal with this topic at greater length after more fundamentals have been laid down, but for now consider that the time-dependent Schrödinger equation, the mathematical basis of the supposed wave nature of particles, holds for all time for all systems, spatial or non-spatial. If Schrödinger’s equation does indeed describe a wave nature of physical objects, then we must accept that these objects are wavelike at all times, not just in certain experimental setups. Of course this cannot be the case, since we in fact observe particles as discrete objects, even in Young’s experiment where they are individually detected at specific points. The result of Young’s experiment and the like can be explained solely in terms of Schrödinger’s equation, so there is no need to introduce a wave nature
of particles, since Schrödinger’s equation is fully consistent with particles existing in discrete form. The spatial wavefunction ψ remains a dimensionless, complex-valued mathematical object. When we determine the wavefunction as a function of position, denoted ψ(x), we are not describing a spatial wave, but projecting the ket vector onto real space, or calculating the product: |x⟩
<x|ψ⟩. The spatial wavefunction ψ itself is not an object in real space and cannot be measured in meters.
Let us examine the composition of the vector |ψ⟩ representing some physical state. |ψ⟩ coexists in an abstract Hilbert space with many other state vectors. We may arbitrarily construct a set of linearly independent vectors to act as coordinate axes (i.e., a basis) of the Hilbert space being considered. For simplicity, we take these vectors to be mutually orthogonal. (Such a set can always be constructed by the Gram-Schmidt procedure.) All vectors in the Hilbert space, including |ψ⟩, may be expressed as a linear combination of the basis vectors |φi⟩, where i is merely an index enumerating the vectors. That is, |ψ⟩ = a1|φ1⟩ + a2|φ2⟩ + a3|φ3⟩ … .
Next, we normalize |ψ⟩ and the orthogonal basis vectors |φi⟩, defining their norms to be equal to 1. This mathematically implies that the sum of the projections of |ψ⟩ onto each basis vector |φi⟩ in the Hilbert space equals the identity operator. (The projection of |ψ⟩ onto |φi⟩ is just the product |φi⟩⟨φi|ψ⟩.) This is nothing more than the closure relation from linear algebra, and it may be generalized for uncountably infinite dimensional Hilbert spaces (such as that of the spatial wavefunction) by introducing Cauchy sequences, a result which can be found in advanced linear algebra texts. Our basis vectors form an orthonormal (orthogonal and normalized) complete set, meaning that any vector in the Hilbert space may be expressed as a linear combination of them.[6]
So far, we have assumed the customary interpretation that |ψ⟩ is a physical state, without specifying anything about it. In practice, however, a physicist comes to know |ψ⟩ by constructing it as linear sum of directly observable states |φi⟩. The |φi⟩’s are defined to be the eigenvectors of the physically observable dynamical quantities we wish to understand. These observables
can be represented as Hermitian square matrices with the dimension of the Hilbert space. Since the matrices are Hermitian, they will have real eigenvalues, as is necessary since we can only measure real values for physical quantities.
The completeness
theorem of quantum mechanics is merely a mathematical statement that the eigenvectors of the observables completely span the Hilbert space. This is an algebraic truism which does not in any way prove that quantum mechanics knows all
there is to know about a system, nor that there is no new physics to be discovered beyond what we can calculate quantum mechanically. In informal physical terms, the completeness theorem says that the eigenstates (states representable as eigenvectors) of the observables span all possible states. All possible states
should not be understood in the broadest sense, but only as applying to those possible states that are distinguishable by the observables (i.e., dynamical quantities) being considered. This completeness does not in any way preclude the discovery of new quantum observables that add to our physical knowledge, as has in fact happened several times since quantum mechanics was rashly declared to be a complete
theory by the Copenhagen consensus. The dimensional extent of the Hilbert space is defined by the observables being considered; hence the completeness theorem provides no deeper revelation than the theorems of linear algebra.
The projections (or properly speaking, the inner products) ⟨φi|ψ⟩, when squared, yield the probabilities of finding each eigenstate |φi⟩. The eigenstates are the only states that can be physically observed. The coefficients of the eigenvectors give the probabilities of being found in each eigenstate, and the measured values of the dynamical variables will always be eigenvalues of the observable.[7]
Setting aside for now the sticky question of when exactly an observation
or measurement
occurs, it is nonetheless clear that we can empirically verify only the eigenstates as real physical states, since they alone have definite real values for observable dynamical quantities. What then of intermediate states, the so-called superposition of states
that supposedly occurs when no one is looking? Typically, physicists follow Bohr in denying that there is any meaning in
asking what happens between observations, yet in the next breath, they
attempt to explain what happens between observations by Paul Dirac’s principle of superposition,
which used the analogy of electromagnetic wave mechanics to suggest that unobserved particles subsist an indeterminate state which is partly in one eigenstate and partly in another. This, it is admitted, is wildly unintuitive, but the empirical verification of quantum mechanics shows that this must be true.
Without denying that there is some sort of physical reality that is characterized by a quantum superposition, I find it to be not only unintuitive but illogical to declare that a thing can at one and the same time be partly in two mutually exclusive states. The analogy with classical wave mechanics will not hold, since there the superposition really is a mathematical combination or summation of two mutually compatible and reinforcing states. A thing can vibrate horizontally and vertically at the same time, resulting (depending on the phase differential) in a combined diagonal vibration. This is not the case with quantum states, which are mutually exclusive and contradictory, since they have distinct eigenvalues for the same dynamical quantity.[8] To allow simultaneous participation in distinct eigenstates would be to admit that a thing can simultaneously be two and three in the same respect at the same time. This defies the principle of non-contradiction at the foundation of linear algebra and indeed of any sane science.
If this were not enough, the the mathematical theory of quantum mechanics itself explicitly recognizes the mutual exclusivity of eigenstates by their orthogonality. For every pair of distinct eigenstates, the product ⟨φi|φj⟩ equals zero. This means the probability of being simultaneously in those two states is zero. The principle of superposition contradicts not only basic common sense, but also the mathematical structure of quantum mechanical theory, which makes the eigenstates orthogonal in probability space. The error of superposition comes from a failure to appreciate that probability space is not ontologically analogous to real space.
It is an understatement to say that the eigenstates are the only states that may be physically observed, as though the superpositions were physically existent yet unobservable. In fact, the eigenstates are the only states with any definite physical meaning at all. Superpositions are primarily mathematical constructs that determine the probability of a system being found in each eigenstate. Treating the superposition as an actually existent physical state is an idea borrowed from classical wave mechanics, where any wave motion may be expressed as the mathematical sum, or superposition, of the normal modes of oscillation. Since the mathematics of the quantum wavefunction is similar in form to that of classical waves, it is tempting to erroneously infer that the physical interpretations must also be analogous. Such an analogy fails, however, since in quantum mechanics the only possible states are the eigenstates,[9] while the normal modes of a classical wave are not the only physically possible oscillatory states. Moreover, the wavefunction does not measure a physical quantity, but a probability amplitude, namely the probability of being found in a particular eigenstate. For the purposes of calculation, a physicist would not pay any penalty for pretending that the superposition is some physical state that exists between observations
or when nature’s eyes blink,
since the mathematical formalism is indifferent to physical interpretation. Nonetheless, this interpretation yields serious philosophical difficulties that are at odds with the principles of noncontradiction and empiricism at the foundation of physical science.
Quantum mechanics upholds the principle of noncontradiction by keeping the eigenstates mutually orthogonal in probability space. Unlike the situation in classical wave mechanics, which deals with real Euclidean space, orthogonality in quantum Hilbert space directly implies mutual exclusivity: ⟨φi|φj⟩ equals zero for all distinct eigenstates |φi⟩, |φj⟩. So a particle can be in one eigenstate or another, but never in two eigenstates at the same time. It is therefore impermissible to interpret a superposition as the simultaneous realization of two eigenstates.
The error of the principle of superposition, as it is commonly interpreted, is to conflate a superposition of potentialities with a superposition of actualities. It is no contradiction to say something is potentially black and potentially white at the same time in the same respect, but it is a contradiction to say that it is actually black and white at the same time in the same respect. The principle of non-contradiction holds of is
and is not
statements, not for is possibly
and is possibly not.
The quantum wavefunction represents possibility or potentiality, not actual physical manifestation, which occurs only in an eigenstate.
Once this is understood, we can appreciate that it is no marvelous accident that we always find natural systems in eigenstates when we test for certain properties. In order to measure or test for a property, we force a particle to give definite manifestation to that property. A fairly strong man may be capable of lifting 100 or 200 pounds, depending on how he is feeling at the moment, but we cannot tell how much he will lift until we put him in a situation where he actually has to lift something. His potentiality in between actions may span the range of lifting 100 to 200 pounds, but when he does lift, it will always be some definite amount, not a range of amounts.
Schrödinger famously posited his cat paradox
to show the absurdity of interpreting quantum superpositions existentially. If the life or death of a cat is dependent on the behavior of a particle in a quantum superposition, we should similarly have to consider it simultaneously alive and dead prior to measurement. Surely, this defies common sense and even the basic scientific presupposition of objective reality in nature.
The common rejoinder to Schrödinger’s cat paradox is to give a mathematical argument to the effect showing that superpositions decohere
on macroscopic scales, when interacting thermodynamically with a large heat reservoir. In this view, the fact that we never observe cats to be simultaneously alive and dead is a mathematical artifact of their large size, not because it is illogical for it to be simultaneously alive and dead. This is an example of empiricism gone mad, for if we were to accept this argument, then we should never raise any purely logical objection to any scientific assertion. Apparently, the law of non-contradiction only applies to certain classes of physical objects. This is nonsense, of course, since the mathematics upon which quantum superpositions and the phenomenon of decoherence is derived is itself dependent on traditional bivalent logic. Decoherence suffices to explain why quantum phenomena are not observed on classical scales, but it does not suffice to establish an existential interpretation of the principle of superposition.
Lastly, it may be contended that superposition states have actually been experimentally observed, as, since the 1950s, there have been numerous claims to have created microscopic or mesoscopic Schrödinger’s cat
states in photons, electrons, and even beryllium atoms. These experiments, however, do not simultaneously detect different eigenvalues for the same observable, which would be absurd and impossible, but rather the presence of a superposition state is inferred by statistical means. In the case of the beryllium atom—actually a 9Be+ ion—the probability of an eigenstate designated |↓⟩ is proportionate to the how often a detection beam causes ion fluorescence. When the ion is in the eigenstate, one photon per measurement cycle is detected.[10] Less frequent detection is considered evidence of a superposition.
There is no fault in these experiments, but only in their ontological interpretation. A particle in a superposition simultaneously has the potential to manifest one or another eigenstate, as is proven by repeated successive measurements. However, it can never actually manifest more than one eigenstate at the same time, so it is inaccurate to characterize the superposition as simultaneous existential participation in two or more eigenstates. An atom, no less than a cat, cannot actually be and not be some determinate property P in the same way at the same time. The law of non-contradiction, being a law of logic, is independent of the subject being considered.
Without the erroneous interpretation of quantum superpositions, there is no need to concoct contrived explanations of how the measuring apparatus interferes with the experiment so that we can never directly observe a superposition. Indeed, none of the theoretical or mathematical foundation of quantum mechanics takes any account of the specific structure of the measurement apparatus. The mathematical formalism of quantum mechanics is nonetheless able to make accurate predictions regardless of the type of apparatus used. Observation
in quantum mechanics does not suppose the existence of a determinate physical apparatus for procuring data.
We do not need to invoke the act of observation to account for why physical systems are always found in eigenstates, which allows us to dispense with another absurdity proposed in some versions of the Copenhagen interpretation (though ultimately rejected by Bohr himself): the idea that by merely knowing the state of a system we alter it physically. This notion is not supported by the mathematical theory of quantum mechanics, which takes no explicit account of the existence of sentient life, nor of the mode by which cognition acts on reality. Just as the mathematics is indifferent to which instrument we use to make an observation, so it is indifferent as to whether the observer is sentient or non-sentient. The collapse of the wavefunction
supposedly caused by the act of observation does not occur in the existential order, but only in the realm of probability or potentiality.
Prior to observation, a quantum system is held to be in some indeterminate state, expressible only in terms of probabilities or potentialities for appearing in one of several possible eigenstates. We have already seen that it is an impossible contradiction for this condition to be an existential superposition of states. It must be in one or another eigenstate, with probability 1, since the sum of the probabilities for the eigenstates is 1, and the eigenstates are mutually exclusive. The problem is that we have no way of knowing which eigenstate it will be in until we actually observe it.
The mere act of observation causes the wavefunction to collapse into a spike, or delta function, about the eigenstate where it is observed. This, however, does not constitute a physical change in the system (i.e., a change in the value of some physical property), since the wavefunction measures probability, not a physical dynamical quantity. The collapse of the wavefunction is nothing more fantastic than a simple corollary of conditional probability. Given that a particle is found in some eigenstate |φ⟩, its probability of being found in |φ⟩ is now 1, and the probability of being found elsewhere is zero. This phenomenon is utterly trivial and common in classical probability (though often misunderstood),[11] and there is no reason to introduce a new interpretation here. Probability is probability, after all, is it not?
Some physics instructors think not, and attempt to distinguish classical probability
from quantum probability,
stating that the former is merely a function of our ignorance while the latter reflects objective physical reality. Both of these interpretations are misguided, though understandably so, as probability can be a slippery concept. The misinterpretation of classical probability is the more fundamental error, making possible the claim of quantum exceptionalism.
In classical statistical mechanics, for example, we are usually taught that there is no real randomness, since the behavior of each molecule is assumed to be fully determined by Newtonian equations of motion. Statistical mechanical phenomena, such as a drop of ink diffusing evenly throughout a liquid without ever reverting to a drop, are considered to be a result of our ignorance of initial conditions. Taken literally, this is obvious nonsense. As Karl Popper wittily observed, even if there was no one to supply the necessary nescience, the result of the experiment would be the same. It happens that the overwhelming majority of possible initial conditions will result in uniform diffusion. The result is caused not by ignorance, but by preparation of the sample in one of the non-special cases.
Similarly, when tossing a coin many times, we might say that the equal number of heads and tails after many tosses is a result of our ignorance of the initial conditions, but this is not correct. It is by preparing the system in a suitable variety of initial conditions that we get the right distribution of results. While, microanalyzing, there is no genuinely
random event, the experiment as a whole yields the results of a random macroevent. What we really have is an exercise in conditional probability. Given a distribution of initial conditions, a certain distribution of final conditions should result.
Further, we observe that statistics has very real, physically measurable implications which can be as deterministic as any classical mechanics problem. Pressure, friction, and temperature, after all, are purely statistical phenomena. Not too many people find statistical mechanics to be philosophically disturbing, since we can be comforted that there is no real randomness at the individual molecular level. However, an ensemble of classical particles could be treated as an object with a wavefunction
that evolves in time as a function of conditional probability. Such a wavefunction would have real physical implications, and could be collapsed
by making suitable measurements.
The quantum wavefunction appears to differ from this classical wavefunction in that it measures an intrinsic propensity
(to use Popper’s term) of the system to be in a given state. Wavefunctions thus represent a sort of neo-Aristotelian potentia. However, this is not necessarily different from the role of the classical wavefunction. In both classical and quantum mechanics, we must assume an initial state or distribution of initial states, from which we derive a probability distribution of final states. In both cases, this distribution of final states may be interpreted as a measure of the propensity to be in each state.
The principal probabilistic distinction between classical and quantum mechanics is that in quantum mechanics we no longer assume determinism on the microscopic level. It is at least theoretically possible that there is a deeper determinism behind apparent quantum randomness, though this possibility has been obscured by unfounded claims of the completeness
of quantum mechanical theory.[12] Nonetheless, quantum mechanics can make accurate predictions independently of any deeper theory, so it may be taken as a fundamentally non-deterministic theory. Still, non-determinism does not provide a logical basis for making a qualitative distinction in our interpretations of classical and quantum probability, insofar as both correlate distributions of initial and final physical states.
The logical and mathematical equivalence of classical and quantum probability can be seen in the use of the so-called density matrix
for mixed quantum states. Suppose we have many particles, of which various percentages are in distinct quantum states (eigenstates or superpositions). This state of affairs can be denoted as an array or density matrix, whose coefficients correspond to the distribution of particles in various
pure states
(eigenstates or superpositions). Physics instructors take pains to impress upon their students that the density matrix is not conceptually the same as a matrix relating superpositions of eigenstates. We are told that the density matrix represents classical probability,
where there is ignorance of which particle is in which state, much as one reaches into a bag of colored marbles. The matrix for a quantum observable, by contrast, is supposed to represent a more palpable quantum probability.
Students can frustrate such pedagogy by realizing that both types of matrices work in mathematically identical ways, and there is no mathematical basis for discerning two different types of probability.
Although there is no qualitative distinction between classical and quantum probability, this does not mean that a superposition is no different from a mixed state. Suppose you have 50% of electrons in the spin up
eigenstate |+⟩ and the other 50% are spin down.
This is not the same as having 100% of the electrons in a superposition. In the latter case, each individual electron has the potential to manifest either of the two spin states, whereas in the former case, each electron is restricted strictly to a determinate eigenstate. This means that a superposition is not to be interpreted statistically; that is, (|+⟩ + |-⟩)/√2 does not mean half the particles are in one eigenstate and half are in another. However, this does not preclude a frequentist interpretation of a quantum superposition, defined by how frequently a particle would individuate one or another eigenstate if an experiment were to be repeated. Likewise, we cannot exclude Popper’s propensity interpretation, mentioned previously.
So far, there is no solid reason compelling us to interpret density matrix probability as any more or less subjective than quantum probability. When we later examine the Young double-slit experiment, we will see that particles in quantum superpositions can affect each other’s probabilistic distribution of outcomes in a way suggesting that quantum probability is a palpable physical propensity, not just a formal quantification of outcome frequencies. We find interference patterns rather than a simple sum of the probability distributions for each particle. This compels us to acknowledge that quantum probability corresponds to some sort of physical reality, but this is not necessarily any less true for classical probability, as is commonly presumed, for the latter can be interpreted in terms of potentialities or propensities. Furthermore, this interference phenomenon is consistent with a frequentist interpretation of probability, as we will show in later examples.
Although there is no need for a new kind of probability to account for quantum mechanics, there are important interpretive distinctions between the classical and quantum wavefunctions. The quantum wavefunction measures a physically real propensity to actualize one or more possible final states, given an initial state (eigenstate or superposition). It is also important to consider that the quantum wavefunction is a function of the system or experiment as a whole, not necessarily of a particular particle or wave. The idea of a ghostly wavefunction accompanying each individual particle may be helpful for some problems, but can lead to confusion in situations like Young’s double-slit experiment, resulting in impossible paradoxes such as travel with infinite speed. These difficulties disappear when the wavefunction is understood to be a function of the entire experiment, and not something that is attached to a determinate body or location.
An important class of quantum paradoxes is choice of measurement, in which the physical state of a system is determined by which dynamical variable the experimenter chooses to measure. At this point, we should clarify what is meant by a measurement in quantum mechanics. Ideally, one may imagine an identically prepared experiment repeated many times on the same particle or system, and due to intrinsic randomness, there may be various outcomes, for which we measure the relative frequency. Equivalently, a measurement can be done with many identical particles or systems simultaneously or sequentially subjected to the same experiment. This latter method is how most experiments are done in practice, though experiments with individual particles have also been done to resolve questions of quantum indeterminacy.
For simplicity, we will consider the two-state spin problem, since all other problems are mere mathematical complications of the same paradox. To measure
spin, we test how particles are deflected by a magnetic field gradient. As demonstrated in the Stern-Gerlach experiment (1922), randomly distributed spin-1/2 particles have an equal probability of being deflected up or down by the same discrete amount with respect to the axis of the magnetic field. For simplicity, we define those deflected up
with respect to the z axis to have positive z-spin, and those deflected down have negative z spin (similarly for the x and y axes). Thus each measurement by a deflecting field forces the spin-1/2 particle, such as an electron, to manifest one of two possible states or outcomes.
Suppose a beam of electrons is sent through a series of Stern-Gerlach measurements. First, the beam passes through a magnetic field along the z axis, with half the electrons deflected up and half deflected down, as expected. Next we take only the electrons exhibiting positive z-spin and put it through another magnetic field. If this field is also along the z axis, we will find only a positive z outcome, as seems intuitive, since our beam now contains only positive z-spin electrons. However, if we insert an apparatus between these two deflectors, with a magnetic field along the x axis, we get a completely different, far less intuitive result. The positive z-spin beam from the first deflector passes through the x axis field, resulting in an even split between positive and negative x-spin. If we take either or both of these beams and pass them through a second z axis deflector, we will get a 50-50 split between positive and negative z axis measurements. This works even for an individual electron, so it is possible for an electron with measured positive z-spin to come out with negative z-spin in the second deflector, simply because of an intervening x-spin measurement!
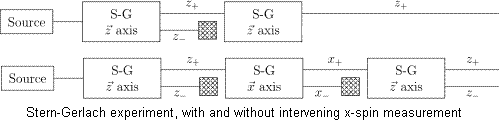
This Stern-Gerlach paradox suggests that what we choose to measure, or the order in which we make measurements, can change the objective physical state of a particle, even to the extent of taking it from one eigenstate to an opposing eigenstate. The x-spin measurement forces the electron to manifest an x-spin eigenstate, which is mathematically expressible as a superposition of z-spin eigenstates. This means the x-spin measurement must at least alter a real physical propensity in the electron, restoring its ability to manifest the negative z-spin eigenstate.
Mathematically, this dependence on the order of measurement is expressible by the non-commutativity of the x-spin and z-spin operator matrices. Once again, we are dealing with ordinary linear algebra, and need only refer to the premises of the mathematical formalism. There is no need to invoke the role of subjectivity, as the accuracy of the mathematical theory does not depend on any determinate definition of subjective consciousness, nor of the design of the apparatus. We only need to specify that each measurement,
observed or unobserved, forces the electron beam to resolve into one or the other eigenstate.
The mathematical formalization of quantum mechanics posits that the eigenstates are mutually exclusive, with zero probability of intersection. This eliminates the interpretation that an x-spin eigenstate is a simultaneous manifestation of both positive and negative z-spin eigenstates. We do not observe the superposition of z-spin eigenstates as such; rather we observe the x-spin eigenstate. We then infer the reality of the z-spin superposition from the mathematical form of the x-spin eigenstate, as well as from the subsequent manifestation of both z-spin eigenstates (after repeated trials) past the final deflection field. All that can be directly measured are the eigenvalues, and no z-spin eigenvalue can be measured by a magnetic field gradient in the x direction.
We do not need to actually observe the x-spin measurement to make this experiment work. We could just skip to looking at the last result, and we would get (after repeated iterations) a fifty-fifty distribution of particles with up and down z-spin. So there are no grounds for explicitly introducing subjectivity into physics here.
Nonetheless, it seems that the very act of measurement
has
forced the particle into an eigenstate of x, and erased any information
regarding z-spin. Here lies the crux of the matter: our measurement
is not really a measurement in a pure intellectual sense of simply coming to know what some determinate quantity is, but rather it is a physical act of filtration that re-orients the particle or system in a manner roughly analogous to how a linear polarization filter acts on unpolarized light, to use Dirac’s famous example. The wavefunction transforms from a z-eigenstate to one of the x-eigenstates in a probabilistic fashion. The electron is always measured to be an eigenstate, but never in a superposition, which is only an inferred state.
It is perhaps meaningless to ask what has become of the z-
spin
just after the x-spin measurement, because at a given time an electron can only manifest one spin, which may be oriented in different
directions by magnetic field gradients. This re-orienting phenomenon is no more remarkable than a polarizer forcing
light to align with it or not pass through at all. That there is in reality only one spin is mathematically acknowledged by the fact that only one of the coordinate spin
operators is necessary to determine the system. Further, two non-
commuting operators may not be simultaneously diagonalized, which is to say
they have no common eigenstates, so we cannot simultaneously measure x-spin and z-spin.[13] It is no mystery that two dynamical variables cannot be simultaneously measured when they are actually different aspects of the same thing. If we placed equal magnetic gradients in both the x and z directions, we would simply align the spin along a forty-five degree angle, so the non-commutativity of operators does not prevent us from observing spin in any orientation whatsoever. It only prevents us from collecting contradictory information, as a particle cannot simultaneously be in an x and z eigenstate.
There are other applications for the idea that non-commutative observables measure different aspects of the same thing. These include orbital angular momentum and quark color, for example. The component operators of angular momentum—Lx, Ly, Lz—all commute with the operator L2, but not with each other. The mathematical interdependence of these operators again suggests that we are dealing with a single dynamical entity of which the component operators reveal different aspects. In quantum chromodynamics, gluon color
is determined by three distinct modalities (redness, greenness, blueness) which are symmetrically interconnected. This makes it possible to model gluon color with a single tensor representing field strength, as can be done in the unification of electricity and magnetism. Just as electric and magnetic fields may be considered different aspects of the same thing, so is the case for the different axes of color states.
This principle of unity in apparent plurality may help us to better understand the famous Einstein-Podolsky-Rosen (EPR) paradox. In this thought experiment, we consider two simultaneously emitted particles that necessarily have opposite spin due to conservation of angular momentum. After they have traveled some distance from each other, we may choose to measure the spin of one particle along some arbitrarily chosen axis. If the other particle, far away, should also have its spin measured along the same axis, we must get the exact opposite result. This, in fact is the case, as has been experimentally verified.[14] One might ask how the second particle could possibly know
to orient itself in the opposite direction as the first, when there is no way of anticipating in advance which axis will be measured. This might be taken to imply action at a distance, where the first particle instantaneously communicates
its measured state to its remote partner. Einstein rejected this possibility not merely on aesthetic grounds (finding it spooky
), but because it would fundamentally contradict relativity by restoring simultaneity at a distance. Einstein has frequently been misrepresented as being unable to come to grips with the unintuitive results of quantum mechanics as if he, of all people, were incapable of accepting new, unintuitive ideas that contradict common sense. In fact, Einstein’s criticism of the Copenhagen interpretation was grounded in the geometry of space-time demonstrated by relativity, as well as a philosophical consistency that many of his peers lacked.
We could resolve the EPR paradox by positing that the entangled
states of the two particles are really different aspects of the same thing. By measuring one of them, we implicitly measure the other. This suggests a degree of holism in quantum physics, which would have been no less aesthetically displeasing to Einstein, but at least would not violate relativity. This interpretation differs from what is commonly taught only in that we do not follow the Copenhagen interpretation of superpositions prior to measurement. On the Copenhagen assumption, both particles are really in some contradictory state until measurement of one particle, upon which the second particle is also forced to change its state, implying action at a distance. In our proposed interpretation, both particles, as a unified system, have only the possibility of manifesting various states. By forcing this system to interact with a magnetic field, it must necessarily exhibit a determinate state. It is not changing from one eigenstate to another, nor from an actual superposed state to an eigenstate, so there is no real physical change or action. We are merely discovering which of the myriad potentialities has become actual.
It may be contended that the determination of an entity’s being is an action of a sort on a metaphysical level. This, however, is not the same as physical action, which requires changes in the value of some dynamical variable. The measurement of a particle does not change the value of any dynamical quantity, but only of the physically dimensionless potentiality represented by the wavefunction. There is no strict violation of relativity, therefore, and the only objection to this sort of action at a distance
is aesthetic, as most scientists have been trained to distrust holistic interpretations as magical.
The proposed interpretation preserves the principle that the only actual physical states are eigenstates, as well as the principle that each physical property has a definite value whenever it is involved in an interaction dependent on that value. In the case of spin, interaction with a magnetic field gradient compels the particle to manifest a definite spin value along the field’s axis. The particle was always there, with the power to exhibit spin in any of various directions, but only a determinate interaction will compel it to manifest one or another of its possible states. Accordingly, there is no reason to abandon the notion that there is objective physical reality between measurements.
An innocent-sounding statement—the particle was always there
—can be decidedly controversial in the context of quantum mechanics, as it contradicts a common interpretation of Heisenberg’s uncertainty principle. We are told that a particle does not have a definite position or momentum prior to measurement, so it is not really anywhere determinate. The Copenhagen interpretation of superposition in this case helps preserve objective reality of a sort, by having the particle smeared out over space between measurements. Yet we have seen that simultaneous occupation of contradictory eigenstates is impossible, and we will show that this is no less true for position and momentum observables. In that case, it would seem that a particle prior to measurement is only potentially here or there, but is not actually anywhere, nor does it have any definite motion or energy. In what sense, then, can there be any objective physical reality before measurement? The Heisenberg principle of position-momentum uncertainty, as well as analogous relations for other variables, will need to be scrutinized carefully, in order to distinguish mathematical necessity from fallible interpretation.
Position and momentum are continuous variables, so they cannot be conveniently represented with matrices and column/row vectors, as was the case with previously discussed observables. We may use the same Dirac notation of bras and kets, but instead of having a discrete set of eigenstates |φi⟩, we have a continuous basis of eigenstates |x⟩ for position and |p⟩ for momentum, which may range over all real numbers, and have corresponding eigenvalues x and p, position and momentum. In other words, there are uncountably many eigenstates and eigenvectors, one for each possible real number value of position and momentum along a given axis. Consequently, these operators cannot be represented as discrete arrays or matrices, but they are still linear and Hermitian, and the probability of being in a given range of eigenstates may be computed by integrating the wavefunction as a continuous function of x or p. There are proofs of the mathematical equivalence of the matrix and integral formalisms, but they would be too cumbersome to include here.[15]
The position-momentum uncertainty principle imposes a constraint on the accuracy of measurement: the more accurately one measures position, the less accurately one can measure momentum, and vice versa. This qualitative assertion comes from the non-commutativity of the momentum and position operators (which is mathematically unsurprising, since momentum is proportional to the derivative of position, and an operator does not commute with its derivative). From the commutation relation of these operators, we may derive that ΔxΔp > ħ/2. Due to the extreme smallness of Planck’s constant (h = 6.626 x 10-34 joule-sec), this relation imposes no practical constraint on direct measurements of position and momentum, which are nowhere near that level of accuracy.[16] We should articulate precisely what this mathematical statement means, and how it differs from some qualitative claims regarding the uncertainty principle. The deltas in the expression represent standard deviation widths of distributions. Recall that the wavefunction tells us the probabilities of occupying various final states given an initial state. The uncertainty principle implies that it is impossible not merely in practice but in principle to determine both position and momentum with infinite accuracy. This may seem like a new paradox, but we have indirectly encountered a similar situation already.
In the two-state spin system, we could have computed standard deviation widths for the spin values prior to each measurement. Had we done so, we would have sometimes found uncertainties as large as the magnitude of the spin (ħ/2). The value of the x-spin would have been completely washed out
prior to the x-measurement, as values of ħ/2 and -ħ/2 were equally probable. This did not imply that spin could never be measured more accurately. In fact, when we do measure spin, it is always in some definite eigenstate. The prior uncertainties are a mathematical consequence of the non-commutativity of the coordinate spin operators, just as the Heisenberg uncertainty principle results from the non-commutativity of position and momentum operators.
Earlier, we proposed that coordinate spin operators may be considered different aspects of the same dynamical quantity. Might we say the same about position and momentum? At first, this supposition may seem dimensionally problematic, but in its favor is the fact that momentum is proportional to the derivative of position, hence the two are inextricably linked. We might continue the analogy with spin, which in turn was an analogy with polarization, and say that a momentum measurement actually forces the position-momentum
to align itself in a certain way. Some physicists do describe all operators in terms of polarization vectors as a convenient mathematical construct, but the polarization metaphor in this case seems far removed from any intelligible physical intuition regarding the dynamical quantities being considered. As noted previously, likeness of mathematical form need not imply likeness of physical interpretation. The relationship between position and momentum is dynamically different from that among the coordinate spin operators, though there remains a fundamental interdependence.
The non-commutativity of position and momentum is not so much a quirk of nature but a mathematical necessity, given that observables are representable as linear operators. (The only physical quirk is the magnitude of Planck’s constant.) All linear operators do not commute with their differential operators, and momentum is necessarily a differential of position, as velocity is the time derivative of position. Classically, position and momentum were treated as independent variables, but operator mechanics brings their necessary interdependence to light. An object can only exhibit momentum by changing its position, and it can only have a definite position to the extent that it does not manifest momentum. Similarly, a particle with narrower uncertainty in its z-spin will have a wider uncertainty in its x-spin, and vice versa. This does not, however, prevent us from observing a definite spin eigenstate. There is no reason to expect differently for position and momentum. We can observe definite eigenstates of each, just not at the same time.
To appreciate the compatibility of the uncertainty principle with the notion that objects can have sharply defined position or momentum (though not both at the same time), we must develop a clearer understanding of the uncertainties
Δx and Δp. Like all quantum wavefunctions, the position and momentum wavefunctions are superpositions of eigenstates, with different probability magnitudes associated with each eigenstate. Since there is a continuum of eigenstates rather than a discrete number, it is convenient to depict the wavefunction as being effectively a continuous probability distribution across eigenstates. The spatial wavefunction ψ(x), for example, can be drawn as a waveform whose absolute amplitude squared equals the probability of being found in a given range of values. The wavefunction is normalized so that the integral area of its absolute amplitude equals 1, meaning the total probability across all possible states equals 1.
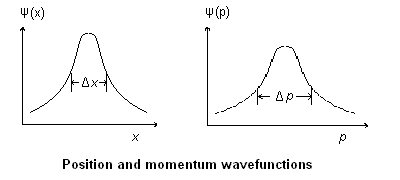
The standard deviation width of a given distribution is denoted by Δx (and likewise Δp for the momentum wavefunction). It is a measure of the distribution or spread of likely eigenstates. This is an uncertainty prior to measurement, just as we do not know what the x-spin of a z-polarized electron will be prior to measurement. When we do make a measurement, we will find our object to be in a definite position. It is a misuse of measure theory to argue that it is impossible to be at a definite point because the area beneath a point is zero. If that were a sound argument, it would prove far too much, rendering continuous space impossible a priori. We must recall that our wavefunction presupposes a continuum of eigenstates, and that we represent it as a waveform only as a necessary convenience to deal with uncountably many eigenstates. There is no basis for abandoning the principle that the only directly measurable states are eigenstates, since these are the only states with real values for dynamical quantities.
The uncertainty principle, rightly understood, does not eliminate the possibility of determining a particle’s position with infinite accuracy in a single measurement. In fact, the act of measurement necessarily forces the object into a position eigenstate, which the quantum formalism defines to be infinitesimal. The uncertainty or waveform width in the complementary momentum function is not infinite (being the Fourier transform of a delta function), so the product of position and momentum uncertainties upon measurement is much less than the Heisenberg limit! There is no contradiction here with quantum mechanical theory. We are only refuting the false interpretation that the uncertainty principle makes it impossible for an individual particle to have a well-defined position upon measurement. Rather, the uncertainty principle is a statement regarding distributions of many particles, or of the same particle in a repeated experiment.
In fact, if we hold this continuous variable formalism to the same interpretive principles used for the discrete case, we should not be able to observe the particle to have anything but a well defined position. Remember that each different position corresponds to a different eigenstate, and all eigenstates are mutually exclusive. This is directly implied by the normalization of the wavefunction to have an integral sum of 1 in probability space. If the eigenstates were not mutually exclusive, the integral in probability space would be less than 1. In fact, one may derive the fact there is an infinite continuum of eigenstates directly from the commutation relation.[17] A particle, therefore, cannot be smeared
in space across multiple eigenstates during a direct measurement of that observable. It may have a probabilistic propensity to be in various locations if repeatedly placed in the same initial conditions, but that is the only sense in which location is indeterminate.
By similar argument, the uncertainty principle does not forbid a particle or system from having a well-defined momentum upon measurement, that is, upon any interaction that is dependent on the momentum value of the particle or system. The fact that a particle cannot simultaneously manifest a determinate position eigenstate and a determinate momentum eigenstate (along the same axis) implies that a particle cannot have a well-defined location at the instant it is engaged in a dynamic, momentum-dependent interaction.
Suppose, as a thought experiment, that we make position measurements of a single particle with infinite accuracy, as is permitted by the formalism of quantum mechanics. Then it would seem, at first glance, that we ought to be able to use two such measurements arbitrarily close in time to measure momentum with infinite accuracy at the same instant. Yet the uncertainty principle plainly forbids this! The uncertainty in momentum, however, cannot be attributable to error in our position measurements, which by assumption are infinitely accurate. The source of uncertainty must be in the measurement of time, which is also the parameter at the heart of the non-commutativity between position and momentum.[18] Momentum is a time derivative of position, and it is this differential relation that prevents the operators from commuting. The uncertainty relation, therefore, reflects an intractable measurement problem between space and time.
Earlier, we posited that non-commuting observables might be considered different aspects of a deeper or more complete physical property. The same may hold in this case, once we see that the position-momentum uncertainty is consequent to the interdependence of space and time in physical dynamics. The idea that space and time are different aspects of the same thing is not novel to quantum mechanics, but is already found in special and general relativity. Reviving our analogy with polarization, we may see a position or momentum measurement as aligning a particle with a determinate direction
in four-dimensional space-time (with each dimension spanning an infinity of eigenstates). To the extent this departs from its previous alignment, its new direction is determined probabilistically, much as x-spin is randomly determined for z-polarized electrons.
It is important to emphasize that the position-momentum uncertainty relation is on a fantastically miniscule scale, on the order of 10-35. No ordinary combination of direct position and momentum measurements even approach this scale. The tiny Heisenberg limit is not the reason for probabilistic distributions of position in electron orbital structure, which is on the much larger angstrom scale (10-10). The scatter plot distributions of electrons bound to atoms are simply collections of many measurements. For each individual data point, the electron is observed to have a well defined position and momentum (even simultaneously, since we are nowhere near the Heisenberg limit). The electron is never observed to be smeared throughout the orbital, and there is no reason to believe this is the case immediately prior to every measurement. We have already noted the incoherence of the unnecessary supposition that a superposition is a simultaneous actualization of contrary eigenstates. In the case of continuous-valued quantum observables, the notion that the wavefunction collapse
is a change in physical states becomes especially problematic. At what point does the smeared particle collapse
into a point? Perhaps when its spatial wavefunction is touched
by the probing particle? Yet the wavefunction extends to macroscopic distances!
If we are consistent in our interpretation of the wavefunction, regardless of whether the observables have discrete or continuous values, it is clear that a particle or system is always in a position eigenstate, a momentum eigenstate, or a superposition. If it is in a position eigenstate, its momentum is not well-defined (i.e., it is in a momentum superposition). If it is in a momentum eigenstate, its position is not well-defined (i.e., it is in a spatial superposition). If it is not in any eigenstate, it must not be interacting with anything, so we can never observe a particle in such a condition, if it ever is so. Since all the fundamental forces are conservative or position-dependent, it follows that particles always have a well-defined position when interacting with fundamental force fields, but not a well-defined momentum, which is why the future positions of particles can only be known probabilistically. This account is consistent with quantum field theory, in which particles are not continuously interacting with fields, but only at discrete intervals. Thus a particle in motion may rapidly oscillate between position and momentum eigenstates, just as an electric charge may interact intermittently with electric and magnetic fields, but not exactly simultaneously.
From the above discussion, it is clear that the uncertainty principle does allow for particles to have definite positions or momenta (though not at the same time), which in turn allows them to have definite though non-deterministic trajectories. If we were to deny this, we would compromise the presupposition of realism in physics, as there would be no determinate physical reality between observations. At most, one could say that an unobserved particle exists
as raw potentiality with no determinations of any dynamic properties. Yet potentiality is contrary to actual existence, so the only sense in which such an indeterminate particle might be said to exist is as metaphysical materia prima, conditioned only by its (generally) unequal propensity to manifest different forms. This may be considered realism of a sort, but it would be firmly outside the physical order. At any rate, quantum mechanics does not require us to abandon physical realism.
The spatial wavefunction gives the probability of a particle appearing at each point in space at some later time, given an initial state. This is true whether we are speaking of a free particle, a hydrogen atom, or a more exotic system. When the wavefunction is represented in time-independent form (in the Schrödinger picture), this is just a convenient way of depicting what the probability distribution would be like an extremely long time after a measurement. The elapse of time is essential, whether it is an explicit parameter or only implied. In free space (i.e., with no external field potentials in the vicinity), the spatial wavefunction over time will look like an ever-spreading wave packet. The particle is not smearing out over space, but only its probability distribution (with respect to its last measured state) is spreading. In a bound atom, the electron’s spatial probability distribution will be defined by well-shaped orbitals, the form of which depends on angular momentum.
Such probability distributions can be interpreted as in classical mechanics: the particle does not smear or become indeterminate, but only our knowledge of the system does. However, this does not imply that the trajectory is deterministic as in classical mechanics. A trajectory can be continuous and well-defined, yet non-deterministic. Conversely, non-determinism does not require us to suppose that a particle smears across space or blinks out of existence between measurements, making a mockery of empiricism.
A denial of the objective reality of particle trajectories leads to faulty interpretations of quantum leaps, tunneling and other effects that would seem to defy plausibility. The common interpretation of these phenomena is along the lines that a particle can really teleport instantaneously from one location to another, or that it is not really anywhere until it is observed. Once more, we must take care to distinguish a faulty interpretation from what the experimentally verified mathematical theory actually says.
The time-dependent Schrödinger equation is a differential equation with a continuous time variable. It holds for all time, during and between measurements. The equation tells us the probability of finding the system in a particular state at some later time, given an initial condition. The probabilistic nature of the wavefunction may demonstrate causal non-determinism, but it is not inconsistent with the idea that a particle is always in a position or momentum eigenstate. Discrete transitions in energy levels do not imply instantaneous motion or blinking on the part of the particle. What may change instantly is the particle’s propensity for being in various locations in the future, so its subsequent behavior will be modeled by a new probability distribution, which is the wavefunction corresponding to the new energy level or state. Quantum mechanics does not justify the effective denial of relativity implied by instantaneously teleporting particles to remote locations. A consistent treatment of Schrödinger’s equation as an expression of conditional probability over time precludes such an interpretation.
The quantum mechanical phenomenon of barrier penetration, where a particle has a finite probability of being found in a finite potential barrier greater than its energy, does not require us to abandon the notion of well-defined particle trajectories. Again, the trajectory may be non-deterministic, but this does not contradict the idea that the particle is constantly in position or momentum eigenstates. While barrier penetration, with its apparent implication of negative kinetic energy, is a non-classical, unintuitive result, we object only to those interpretations of quantum mechanics that are strictly illogical, contradictory, or incoherent.

In the type of barrier penetration known as quantum tunneling, a particle appears to move from one potential well to another, passing through a finite potential barrier where its kinetic energy would seemingly have to be negative. Some physics texts and instructors, in view of the impossibility of negative kinetic energy, say that the particle must instantaneously jump from one well to the other side of the barrier.
Yet experiments have shown that tunneling electrons can be observed in transit through a barrier, with positive kinetic energy. Those who acknowledge this fact usually attribute the positive kinetic energy to energy added by the act of measurement,
with the usual Copenhagen ambiguity as to whether we are speaking of the peculiarities of the measuring apparatus or the mere act of knowing the particle to be in a definite state. We certainly should address the problem of whether the kinetic energy is well-defined at all times, though even if it is not there could still be a well-defined, non-deterministic trajectory.
It is asking far too much to expect that any measuring apparatus whatsoever will always affect a particle in just such a way as to give it a conveniently positive kinetic energy. If physics is to remain a fundamentally empirical science, we should accept that particles in the classically forbidden
region always have positive kinetic energy, since they have always been observed as such. The idea that they suddenly acquire this quality as the result of observation, while technically irrefutable, has no basis in experimental observation, nor is it indicated by the Schrödinger equation.
Some suggest that the act of observing position confines the particle, thereby increasing the spread in its momentum (per Heisenberg’s principle) to yield a positive kinetic energy (which is proportional to momentum squared). This is a physical misinterpretation of a mathematical fact. Detecting a particle at some definite position imposes a condition on the probability distribution, or collapse of the wavefunction.
The position wavefunction is narrowed to a spike while the momentum wavefunction is spread or flattened. Yet this change in probability distribution is not a change in actual momentum, but is an indicator of increased future variability in momentum. The Heisenberg principle is independent of the choice of apparatus, so it is really a statement that being in a definite position eigenstate is fundamentally incompatible with manifesting a definite momentum, due to the interdependence of position and momentum. This interdependence persists regardless of whether the particle is being measured, and at any rate the Heisenberg limit for distribution spreads is much smaller than the scales relevant for experimentally observed barrier penetration.
If we plot the ground state momentum wavefunction of our proposed particle at any point in time, it will show the momentum to be non-negative everywhere. Thus the kinetic energy T of the particle is always non-negative, according to formal quantum mechanical theory, even though it is capable of barrier penetration in the ground state. Therefore, barrier penetration cannot be generally interpreted as implying negative kinetic energy. Rather, we should say that quantum barrier penetration or tunneling implies a non-zero probability of having a positive kinetic energy in an area where that would be classically impossible. The fact that the total energy eigenvalue E is less than the nominal potential V would be a contradiction classically, but we know that the classical potential is arbitrarily defined and illusory as a physical entity (with no meaning, for example, at relativistic energies), so we perhaps should not be too surprised to discover that E = T + V is not a universal law, and that only infinite potential barriers are absolutely impenetrable.
In short, the observation of barrier penetration or quantum tunneling does not contradict the idea that particles have definite trajectories. Tunneling particles are actually observed in transit with positive kinetic energy. Although this contradicts the classical relationship among energy, kinetic energy and potential, it is entirely consistent with the mathematical theory of quantum mechanics for a particle to have positive kinetic energy inside a potential barrier even prior to observation.
The time-energy uncertainty relation is only superficially analogous to the Heisenberg uncertainty principle. This uncertainty relation is often called non-canonical
because it does not result directly from the non-commutativity of operators, since time is merely a parameter, not an operator, in quantum mechanics. Instead, the relation is a direct consequence of the mathematical form of the time evolution operator: eiEt. This operator defines how the wavefunction propagates over time per the (time-dependent) Schrödinger equation. From the time evolution operator, we may derive the uncertainty relation: ΔEΔt > ħ.
The uncertainty
in energy, denoted ΔE, refers to the standard deviation of a statistical distribution of energies of identically prepared systems, not to a superposition of energy states for a specific system. The quantum
in quantum mechanics comes from the discovery that we only observe particles in discretely quantized energy states, whose energies are eigenvalues. It would be inconsistent with this realization to interpret ΔE, which is generally non-zero, as signifying that the particle is rarely or never in a specific energy state. Note that our statistical interpretation of ΔE is fully consistent with how we treated Δx and Δp. The Copenhagen interpretation of the uncertainty principle, by contrast, would arbitrarily give the operators x and p a totally contrary ontological treatment than what we have seen is needed for E.
Δt is the characteristic time,
which defines the average frequency with which the system changes between eigenstates of some observable A. Recall that eiEt is the time propagator for some wavefunction, and a wavefunction has to be defined with respect to some observable. So ΔEΔt > ħ means that, the more frequently the system changes between eigenstates of A (i.e., smaller Δt), the greater will be the variation in observed energy levels. It only stands to reason that a rapidly changing system will have a less stable energy state, while a slowly changing system is more likely to remain in the same energy state.
The time-energy uncertainty relation is a purely mathematical or statistical relationship between the characteristic time and variation in energy states. It is not reflective of a physical interdependence between time as such and energy. It is wrong to say, The more accurately you know the time, the less accurately you know the energy,
for time is not an observable. We should not, then, treat the time-energy uncertainty relation as analogous to the Heisenberg principle, a judgment with which even Copenhagen theorists can agree. We depart from the Copenhagen interpretation only in our consistent insistence that the only actual physical states are eigenstates. Superpositions represent only tendencies or propensities to actualize one of several possible eigenstates, yet the only physically manifest state (i.e., that which is observed
by an interaction that compels the determination of a physical property) will be a definite eigenstate with a measured eigenvalue. This is true for all observables, regardless of whether they have a discrete or continuous set of eigenvalues.
I repeatedly stress that the eigenstates are the only physically actual states, so we should not stumble into the Copenhagen error of treating continuous eigenstates in a way that contradicts what has been established for discrete eigenstates. Dirac, for example, in his discussion of position-momentum uncertainty, astonishingly maintains that a system may never be in an eigenstate of position, nor of momentum. We must surely acknowledge that a system cannot be in simultaneous eigenstates of position and momentum (on the same axis), but Dirac makes the further claim that the system cannot even be in an eigenstate of one or the other observable.[19] On what basis could this claim be made?
The non-commutativity of x-spin and y-spin does not contradict the principle that we can always measure the x-spin to be in an eigenstate, and similarly for the y-spin; it only prevents us from measuring both to be in an eigenstate at the same time. So the non-commutativity of operators cannot be invoked as a justification for Dirac’s interpretation. The only other consideration would be an inexplicable abandonment of the full equivalence between continuous and discrete systems, made possible only by the fact that we cannot, of course, measure anything with infinite precision, so we are unable to test whether or not systems truly manifest eigenstates that vary infinitesimally. Yet if we deny that a system can ever be in a position or momentum eigenstate, we would have a gross incongruity, interpreting the continuous case as never being in an eigenstate when measured, while interpreting the discrete system as always being in an eigenstate when measured, even though they are fully mathematically equivalent.
Continuous eigenstates are all mutually orthogonal in infinite-dimensional Hilbert space, and their inner product is a Dirac delta function, which equals 1 when the eigenstates are identical, and 0 if they are different by even the tiniest variation. As in the discrete case, the continuous eigenstates are mathematically defined to be mutually exclusive possibilities, so it is inconsistent to treat their superposition as simultaneous actualization of contrary eigenstates. At any rate, there is certainly no reason to deny that the system is an eigenstate when it is measured. Recall that measurement in quantum mechanics can be treated in a purely formal sense by applying the observable’s linear operator to the wavefunction. Such application gives us a definite eigenvalue, which is why we can subsequently describe the wavefunction as momentarily collapsed
into a delta function or spike at one specific eigenstate.
If, on the contrary, we supposed that a measured system is never in an eigenstate, then measurement could never give us a definite value for position or momentum even in principle. Such a supposition is flatly contradicted by the mathematical formalism, which allows—in fact, requires—the measured system to produce a definite eigenvalue corresponding to a single eigenstate. If this had not been the case, we would have to say that a system simultaneously exhibits different—i.e., contradictory—values in the same act of measurement. Such physical and philosophical absurdity, far from being a mark of profundity, is an illogical inference from quantum mechanical theory, made necessary only by a flawed ontological interpretation.
The ontological interpretation of eigenstates needs to be consistent for discrete and continuous observables. This necessity is further evidenced by the fact that often the only difference between the discrete and continuous case is the mere presence or absence of a potential. If the system is always in a (discrete) eigenstate when a potential is applied, it is hardly consistent to say that it is never in an (infinitesimal) eigenstate when the potential is removed. Further, the potential itself depends on relative positions of system components, so if these positions are ill-defined, so is the potential, and even the energy for that matter, though we know in fact that energy has well-defined values. We have already seen that the spread in an atomic orbital wavefunction is not a measure of an actual uncertainty or smearing
in an individual position measurement. It is inconsistent to suppose that this should suddenly become the case for a free particle, especially since there is no sharp distinction between bound
and free,
as particles are generally influenced by some potential, however small, at all times.
It may be argued that all I have said is just irrelevant quibbling over philosophical interpretation, as there is no empirical test that would clearly favor this or the Copenhagen interpretation. To an extent, this is certainly true, since much of what is in dispute is what is reality in between measurements. However, it is self-defeating for physicists to exhibit their usual contempt for metaphysics, unless they wish to disavow the claim that physics has something to say about objective reality. Although empirical tests are an essential part of natural science, it is no less essential to have a sound theoretical structure and credible pre-theoretical suppositions. Physics is especially dependent on mathematics in order to give its theories form and logical structure, and it is none the poorer simply because mathematics is abstract and ethereal. Physics also has metaphysical assumptions it takes for granted, and it is not a quibble or irrelevant matter when physicists start to interpret a particular theory in a way that contradicts the necessary presuppositions of physical science. Denials of the principle of non-contradiction or of objective reality ought to concern physicists no less than philosophers, as these logical and metaphysical claims are presupposed by physics. If physicists are concerned with understanding natural reality, as opposed to merely making quantitative predictions, the correct ontological interpretation of quantum mechanics cannot fail to be a matter of great concern.
Metaphysics is especially unavoidable in quantum mechanics, since this theory deals explicitly with the transcendence of determinate physical states. The metaphysically simplistic idea that existence is the only sort of being will no longer hold up when faced with quantum superpositions. Yet the usual attitudes of physicists toward mathematical objects are ill-equipped to handle such a problem. We are taught that some theoretical objects are purely mathematical and non-physical, so they should not be treated as physically causative agents. Other theoretical objects, such as fields, are held to be physically real, on account of the fact that they have observable physical effects. The quantum wavefunction, however, fits neither of these descriptions. It is a non-physical object that nonetheless has real physical effects, which contradicts the common assumption of metaphysical naturalism, where only palpable physical objects can produce physical effects. At a loss, most physicists inconsistently oscillate between interpretations of the wavefunction, which is alternately conceived as a probability calculator or as a real ghostly entity that accompanies a system and spreads it across physical states. Some, like Bohm, have gone to the more consistent extreme of always treating the wavefunction as a definite physical object, in order to restore strong determinism in physics. Yet neither strong determinism nor metaphysical naturalism are as fundamental to physics as belief in objective reality and the law of non-contradiction. It is these tenets I have sought to preserve with the ontological interpretation presented here, not because I have some aesthetic attachment to these principles, but because rational thought demands them.
The quantum wavefunction makes the dependence of physics upon metaphysics (and mathematics) physically explicit, so it can no longer be pushed into the background. The wavefunction captures the raw potentiality that precedes—not just temporally, but metaphysically—determinate physical activity. Attempts to treat the wavefunction itself as a determinate physical object are doomed to incoherence, since treatment of superpositions as simultaneous actualization of contrary states is a logical contradiction. Instead, we must acknowledge that the wavefunction is something that does not fit in the simplistic object-property ontology assumed by many physicists, mathematicians, and computer scientists. It is something, but it is not a determinate object nor is it a property of a determinate object.
Probability is the only link between the quantum wavefunction and physical reality, so interpretation of the wavefunction depends on our ability to correctly interpret quantum probability. We have seen that even in classical physics, probability, properly considered, does not imply that outcomes are dependent upon ignorance or knowledge. Rather, outcomes are determined by the actual distribution of initial conditions. The same is true in quantum mechanics. Strong determinism, as is found in classical mechanics, is just the special case where a certain initial condition produces the same result, or eigenstate, with probability 1. (We might say that all eigenstates are stationary in a deterministic system.) If we understand probability in general as a distribution of initial states leading to a distribution of final states, without assuming anything else, we have a framework in which all probabilistic phenomena can be explained with full equivalence.
The false distinction between quantum and non-quantum probability was invented by twentieth-century physicists who, having previously held strong determinism as a fundamental fact, believed that only a fundamentally new concept could suffice to overturn this view of the world. In fact, as we have seen, they might have altered their view even before the advent of quantum mechanics, if they had understood classical probability properly instead of regarding it as a mere computational device. Rather, all objective probability distributions, quantum or non-quantum, are measures of the real propensity of a system to reach a final state given a distribution of initial states.
It may be complained that terms such as ‘potentiality’ or Popper’s ‘propensity’ are unacceptably vague and therefore add no content to our knowledge. If we know that they do not mean actual, determinate existence, we already know much more than the Copenhagen theorists. Logical consistency and coherence are not modest gains. Still, we may try to develop these concepts a bit, without veering off into a subtle metaphysical discussion. First, potentiality implies something more than mere possibility. A thing is metaphysically possible if there is no metaphysical principle that forbids its existence and none that requires it to exist; thus there are no degrees of possibility. By potentiality, we are speaking of a real power to bring something into existence. There can be different orders and degrees of potentiality. ‘Propensity’ is a quasi-teleological way of speaking about a certain potentiality, describing it as a tendency or inclination to actualize one state rather than another. The concept of propensity is especially useful in quantum mechanics, where the objective probabilities are different for various possible states, implying real tendencies or inclinations toward more probable outcomes.
The need for metaphysically sound interpretation can also be seen in the treatment of virtual particles. Like the wavefunction, virtual particles are mathematical constructs with real physical implications. They are intermediate steps in quantum calculations of particle interactions. The supposed proof of their real physical existence is that the endproducts of interactions are accurately predicted by models postulating their intercession. Yet the same could be said of many models that use mathematical objects not corresponding to physical objects. Again, the physicist who insists that something with physical implications must itself be physical will fall into incoherence. He is forced to make the anti-empirical assertion that a virtual particle conveniently blinks in and out of existence before it can be measured. Yet the formalism dictates that virtual particles cannot be measured in principle since they are not eigenstates of the full Hamiltonian. The formalism is indifferent to the nature of the measuring apparatus, so the unmeasurability of virtual particles goes well beyond any practical limitations. Rather, virtual particles are fundamentally unobservable, which is the same as saying that they never become actual.
The usual excuse for not observing virtual particles is that the characteristic time of the transition between initial and final states is extremely small, so there is not enough time to observe the virtual particle. This is a terrible misunderstanding of the characteristic time, which is not a measure of how long a state transition or interaction takes, but rather is a statistical quantity that measures the expected amount of time, on average, that a system will remain in a certain state before changing to another state. The characteristic time is something which can be measured only by the consideration of many identically prepared systems. The change in eigenstate for a given system is (by formal assumption) instantaneous, as it merely entails adopting a new wavefunction and behaving accordingly thenceforth, so there is zero time for the intermediate virtual particle to actually exist. Virtual particles never exist in time, but rather they represent intermediate metaphysical causes in particle interactions. The real causal efficacy of virtual particles compels us to abandon the conventional notion among physicists that causality is always temporal, a thesis that competent metaphysicians already know to be false.[20]
To illustrate more clearly the principles of ontological interpretation we have outlined, let us now reconsider Young’s double-slit experiment. We begin with a single photon, a corpuscular entity that is associated with an electromagnetic field. The field propagates in a wavelike manner, but this electromagnetic wave is not, properly speaking, the wavefunction of the photon, which is similar only in mathematical form. The wavefunction is a function in probability space (more accurately, Hilbert space), which gives the likelihood of various outcomes given specified initial conditions. In particular, we are concerned with the spatial wavefunction of the photon, which determines the probability of its future position. The wave-particle duality exhibited by the Young experiment is not between the corpuscular photon and its electromagnetic wave, but between the photon and its spatial wavefunction. We could avoid this confusion by using an electron instead of a photon in the Young experiment, which will give similar results.
First, let us specify that our photon or electron has a specific position at a given time. This necessarily implies ambiguity in its momentum, per the non-commutativity resulting from the fact that momentum is a differential of the position operator. This momentum uncertainty means the future trajectory of the particle is non-deterministic. Yet the Δx and Δp of the uncertainty principle are statistical spreads obtained from measurements of many identically prepared systems, not descriptions of an individual photon or electron. This is confirmed by the fact that we observe each individual photon or electron to hit a specific point on a photographic plate, and we only see a spread of positions when we measure many such particles. As the photon or electron passes through a single slit, the uncertainty Δx of its final position on a photographic plate (placed at some large distance from the slit) is much greater than the localized mark that the individual photon will leave. A large positive value for Δx after the particle passes through the slit clearly does not indicate that a particle is smeared across space. There is no reason, then, to interpret Δx as implying a spatial smearing of the free particle prior to approaching the slit, though the spatial uncertainty in that case is too small to be experimentally verified.
Let us repeat our preliminary single-slit experiment with many identically-prepared particles, and acquire a statistical distribution of position measurements on the photographic plate, from which we can construct the spatial wavefunction ψ(x)(or confirm its calculated form). Each individual photon or electron behaves in a corpuscular manner, albeit in a probabilistic way which is different from the billiard-ball determinism of classical mechanics. No matter where we place the plate, any distance before or after the slit, each particle leaves a tiny local imprint. It is utterly anti-empirical, and therefore unscientific, to maintain that the photon or electron conveniently delocalizes
when it is not being measured. This is akin to saying there is a fairy on your shoulder who disappears whenever you turn to look. We always observe individual particles to have definite locations in the Young experiment, so we should favor an interpretation that is consistent with the idea that particles have definite yet non-deterministic trajectories.
When there are two slits, the resulting spatial distribution of photons or electrons on the photographic plate resembles that of interference fringes in electromagnetism, even when we emit only a single particle at a time. With an ordinary electromagnetic interference pattern, however, we are measuring the amplitudes of actual electromagnetic fields that constructively or destructively interfere with each other. In Young’s experiment, by contrast, we are measuring a probability amplitude via a statistical ensemble of photon (or electron) intensities (i.e., the number of particles landing in each spatial interval). Instead of physical electromagnetic fields interfering with each other, Young’s experiment shows a mathematical interference
of wavefunctions. What disturbs our intuition is that the two-slit interference pattern is not the mere summation of two single-slit patterns, as we would expect if each photon were passing through one slit or the other. It is difficult to see, on the assumption of each particle having a definite trajectory, how its behavior could be affected by the presence or absence of another slit some distance away from the one through which it is passing.
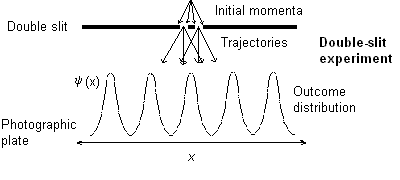
Here it is necessary to reiterate that a wavefunction, like any other probability function, is meaningful only if initial conditions are specified. For the double slit experiment, we have a random distribution of photons, most of which will not pass through either slit, and some of which will pass through one or the other slit. The wavefunction is not some ghostly companion of the photon, but a function of the experiment being considered; any failure to specify initial conditions will change the wavefunction’s form. The configuration of the slits is relevant to the computation of the wavefunction of the experiment. No individual particle physically interferes with any other particle, for the experiment can be conducted one particle at a time. Each photon (or electron) passes through one or the other slit, but its probability of final destination is a function of the system as a whole, not just the part that this individual photon happens to interact with. The same is true of a ball falling down a pinboard; if a pin is removed, the ball’s probability distribution of final positions instantly changes, even if it does not pass through the area of the missing pin. The change in distribution will be measured through repetition, as some balls will be affected by the missing pin. Yet we are still left with an apparent discrepancy: why isn’t the double-slit wavefunction the mere mathematical summation of two single-slit wavefunctions?
For the double-slit experiment, we can only specify at best some narrow range of initial positions and momenta for the photons. If we were to make the starting conditions of all photons identical up to the Heisenberg limit(a practical impossibility), they would all pass through the same slit (given that the slit distance is large compared to the Heisenberg limit), and we would essentially have a single slit experiment. As the double-slit experiment is actually conceived, the total distribution of incident photons will be some cone of momenta approaching the region of interest. This distribution of momentum states may be treated mathematically as a wave. Schrödinger’s equation is mathematically similar to the classical wave equation, which is at once a pleasant and lamentable discovery. The advantage of mathematical elegance is mitigated by the fact that the wavefunction in the Schrödinger equation is liable to be confused with the electromagnetic wave or a tangible mechanical wave.[21] This confusion is especially likely when the particle is a photon, since the probability distribution of position outcomes corresponds to the energy density of the electric and magnetic fields. Yet it is not the electromagnetic wave as such, but rather the wavefunction that is responsible for the form of the probability distribution, as is proved when we use an electron instead of a photon. It is the mathematics of the Schrödinger equation’s wavefunction-derived probability distribution that leads to the interference-style fringes.
Per the ergodic principle of probability, it is irrelevant whether the two-slit experiment is done with many photons or one photon at a time. Thus we find the interference-style probability distribution even when one particle at a time is passed through the double slit. It is clear that this is not a matter of photons interfering with one another, yet Dirac’s inference that the photon interferes only with itself
is unjustified.[22] The similarity with electromagnetic interference is purely mathematical. Our probability distribution is derived from the form of the wavefunction, not from an electromagnetic wave. Uncertainty in the initial momentum makes possible passage through either slit, so the entire range of possibilities from passing through either slit must be included in the wavefunction. The mathematical form of the distribution of outcomes results from the fact that the initial distribution of momenta may be modeled as a wave, giving us a classical interference pattern. No actual physical object is interfering with itself or anything else. We might speak of the wavefunction as mathematically expressing interference between two sets of states, but the wavefunction is not an actual photon, only a measure of future probabilities.
The wavefunction, nonetheless, measures a physically real potential for actualizing various possible states, so any interference
among possibilities is something more than a pure formality. Here it is explained in terms of the distribution of permitted initial states, which collectively behave (as potentialities) in a wavelike manner. As long as our photons (or electrons) are randomly sampled from the allowed distribution of initial states, we may expect them to have the resulting interference distribution. Shortly before it approaches the double-slit, the future momentum (both magnitude and direction) of the photon is necessarily indeterminate enough to permit passage through either slit. These various possible momenta might be said to interfere
with each other only in the sense that they collectively constitute a wave-mechanical propensity. Yet there is no interference for each individual photon as it passes through one or another slit. The interference is entirely on the order of potentiality.
We must recall that the spatial wavefunction in the Young experiment is a combination of eigenvectors in infinite dimensional Hilbert space, with each dimension corresponding to a real or imaginary component of a position eigenvector. The spatial wavefunction is not a function in real space, though it may sometimes be convenient to treat it as such, and there is of course a mathematical relation between points in real space and their corresponding eigenvectors. There is no basis, then, for interpreting a superposition as the simultaneous occupation of different points in real space, as discussed previously. This erroneous conception of superposition as simultaneous actualization is the cause of Dirac’s claim that the photon interferes only with itself,
being partly in two different states (going through one or the other slit). Each photon, it is true, has the very real physical possibility of going through either slit (owing to the uncertainty in its momentum upon approach), but it actually only goes through one or the other. This is indicated by the fact that no matter where we place the photographic screen, we always observe the photon at a determinate point. It is groundless and anti-empirical to assert that the photon smears out into a spatial wave only when we are not looking, regardless of when we decide to look.
The erroneous conceptualization of superposition forces physicists to adopt an effectively subjectivist view of physical reality, in sharp contrast with their ordinary opinions, and indeed with all other results of experimental science. Schrödinger indicated this absurdity with his cat paradox, which attempted to connect quantum mechanics to commonsensical reality. At what point does a cat whose life or death is decided by a quantum superposition cease to be both alive and dead? That which is logically absurd at the macroscopic level must also be so at the microscopic level, regardless of whatever other differences may abound. Unfortunately, physicists are so loath to regard philosophical problems as serious considerations, that they are even willing to sustain the absurd belief that logic depends on physical scale. This contempt for philosophy has made it necessary to point out that conventional interpretations of quantum mechanics are also internally inconsistent, for if physicists will not respect philosophy, perhaps they will at least respect the mathematical concepts at the base of quantum mechanical theory.
Most physicists would recoil at the thought of injecting metaphysics into physics, even though this may be a practical necessity. If this is too much to accept, perhaps at least they can be persuaded to interpret quantum mechanics in a way that is consistent with empiricism and with the mathematical concepts underlying the theory. We have already noted some internal inconsistencies that might be easily remedied.
Arguably the most remarkable inconsistency in the Copenhagen teaching is its refusal to give the eigenstates of continuous observables (e.g., position and momentum) the same physical interpretation that is given to eigenstates of discrete observables. In the discrete case, it is strenuously asserted that a quantum measurement always results in an eigenstate, yet in the continuous case we are told that a system can never be in an eigenstate, even in principle. These diametrically opposing interpretations are at odds with the fact that the discrete and continuous systems are mathematically proven to be fully equivalent. Both continuous and discrete wavefunctions are vectors in Hilbert space, such that the modulus squared of their inner product with each eigenvector gives the probability of occupying that eigenstate. The eigenstates are axiomatically defined to be mutually exclusive and non-overlapping in probability space, and their combined probability is 1, leaving zero probability for any non-eigenstate. The uncertainty principle for continuous observables is not a sound argument against the physical reality of eigenstates, for we can construct analogous uncertainty principles for discrete observables, while still admitting that measurement always yields an eigenstate.
While we see above how similar things are treated differently, there is also the opposing error of treating different things as though they were the same. The most egregious example of this is interpreting the wavefunction as though it were an actual physical wave. The mathematical definition of the wavefunction in Hilbert space positively precludes this interpretation, yet it persists because of the confused notion that anything with physical implications must itself be a physical entity. Treating the wavefunction as physically actual results in the erroneous interpretation of superposition as manifesting contradictory events, in defiance of what the mathematical formalism explicitly defines (the inner product of non-identical eigenvectors being zero). If you are going to make assertions that contradict classical Aristotelian logic (with its laws of non-contradiction and the excluded middle), you had better ground it in a mathematical formalism that does not presuppose such logic. Yet quantum mechanics does not rely on any exotic alternative such as Heyting algebra, but rather it is grounded in ordinary linear algebra. All quantum observables are modeled as Hermitian operators, which conveniently have real eigenvalues, since we can only measure something to have a real value. Any attempt to interpret quantum mechanics in a way that contradicts classical logic is not grounded in the mathematical theory, but actually opposes it.
If physicists do not wish to become philosophers, they should at least be consistent in this avoidance and let go of any philosophical thesis that is not essential to their discipline. One such thesis is the idea that whatever affects experimental results must itself be physically actual. It is possible to conduct physics without this assumption, but physics is not possible without traditional logic. In the face of the realities presented by quantum wavefunctions, this thesis can no longer be sustained except by openly contradicting classical logic in the ontological interpretation of superpositions as physically actual. We need logic, but we do not need the thesis, so we should discard the latter. However you wish to understand potentiality or propensity, if you care to address the question at all, it must be sustained that this is not the same as actual existence.
It is easy to disprove the claim that the Copenhagen interpretation is vindicated by the repeated confirmation of the mathematical theory. We need look no further than to the fact that the mathematical theory still admits a broad range of interpretation even among so-called Copenhagen theorists themselves. What has been vindicated is non-determinism over determinism, and non-locality over locality, yet both these dichotomies can admit various interpretations. When we say something is indeterminate, we may mean that its future state cannot be predicted by the present, or that it is simultaneously in many states, or it is not in any particular state, or it is potentially in many states, but actually in no particular state. When we say something is non-local, we may mean that its behavior is affected instantaneously by some distant event, or that it is simultaneously in many places, or that it is nowhere in particular, or that it is part of a whole whose state is determined by measurement of any part.
Most attacks on the Copenhagen interpretation by physicists have come from the standpoint of trying to restore strong determinism and causal locality. This is a futile attempt to resuscitate nineteenth-century-style materialism, which still has a strong following for reasons of philosophical and anti-religious preference. With the exception of the unfalsifiable many-worlds
interpretation, the deterministic and locally causal alternatives have all failed to convince many physicists, and with good reason, considering the wealth of experimental evidence against determinism and locality. Yet even Copenhagen theorists are reluctant to abandon the central tenet of materialism: the idea that stuff
is ontologically fundamental. Quantum mechanics makes nonsense of materialism, as it posits a wavefunction of potentialities that is metaphysically prior to any determinate substance, and this wavefunction may even determine system states non-locally. The only way to preserve a materialist worldview is to treat the wavefunction as though it were stuff,
which is in fact what Copenhagen theorists do, albeit inconsistently, while Bohm asserted the wavefunction’s physical existence unequivocally. Treatment of the wavefunction as actual, however, leads to a host of contradictions, such as we have discussed. We do not need materialism in order to have physics, so materialism must also be discarded, in the face of the experimental evidence and the need for a logically consistent theory.
The failure of deterministic and causally local alternatives to the Copenhagen interpretation has led to a false sense of confidence in the latter. Yet many aspects of this interpretive scheme, such as Dirac’s notion of superposition and the subjectivist understanding of Heisenberg uncertainty, are not logically required by the mathematical formalism, nor are they experimentally verified facts. There are in fact more cogent alternatives, such as the propensity
interpretation of the wavefunction advanced by Popper and the variant offered in this essay. Such interpretations are possible only when we properly understand the probabilistic, non-existential nature of the wavefunction, and apply this concept consistently in all experiments, rather than resort to the ad hoc interpretations used by Copenhagen theorists.
Since the Copenhagen interpretation is not consistent in how it treats the ontology of the wavefunction and the subjectivity of probability, it forces us to accept multiple paradoxes
of disparate qualities. This is in striking contrast with relativity, which requires us to accept only one non-intuitive thesis, the invariance of the speed of light, from which all else follows logically. Instead, quantum mechanics is replete with ad hoc interpretations that follow no general scheme. Sometimes we say the act of measurement perturbs the system. At other times, we say reality is intrinsically ill-defined, or the question has no meaning. Countless physics students have muddled along in this inconsistent interpretive scheme, supposedly learning to think quantum mechanically,
while in fact accepting the least offensive absurdity in each scenario, and applying the formalism mechanistically to yield the correct results. Richard Feynman at least was honest enough to admit, No one understands quantum mechanics.
However, this does not mean that it cannot be made understandable.
A more sound understanding can begin by accepting the dependence of physical events on a metaphysical potentiality known as the wavefunction. Once the stumbling-block of materialism is finally set aside, the path is clear for a conceptually coherent formulation that can be applied consistently to all scenarios in quantum mechanics. I have drawn the outline of such a formulation in this essay.
Physics has been able to progress admirably as a science despite the qualitatively poor interpretations of its theories. The great danger in Copenhagen sophistry is not that it will harm physics as a discipline, but that it leads to egregious errors in other disciplines, which accept the Copenhagen interpretation with the authority of scientific truth. Countless books and articles have invoked quantum theory as proof that reality is subjective, or that the act of observation changes physical reality, where ‘observation’ is often considered to mean sentient perception. The Copenhagen theorists have sown fertile fields for solipsists and nihilists of all varieties, as well as for their more temperate agnostic brethren. Although some of these gross philosophical misinterpretations have been corrected within the physics community, the misconstrual of quantum mechanics by the outside world is likely to persist much longer. Nonetheless, it may be reasonable to hope that the current subjectivist quantum quagmire will someday be perceived as an historical curiosity, much as we now view those philosophically innocent materialists of the nineteenth century.[23]
See also: Locality, Determinism and Bell Tests | Introduction to Ontological Categories
[1] Heisenberg, Werner. Physics and Philosophy [1958] (New York: HarperCollins, 2007), pp. 15, 27, 134, 154-5.
[2] I do not count the many-worlds
interpretation as ontologically distinct from the Copenhagen interpretation, insofar as it describes this world. Its sole function is to restore strong determinism to physics on a meta-cosmological level by making all possible paths actually followed, even if it is by the decidedly unparsimonious, unfalsifiable supposition that there is an uncountable infinity of universes.
[3] The names ‘bra’ and ‘ket’, coined by Paul Dirac, are derived from the fact that they denote the left and right halves of the bracket
defining the norm.
[4] For example, if |ψ⟩ = a|α⟩ + b|β⟩, then the probability of |α⟩ is |a|2, and the probability of |β⟩ is |b|2.
[5] A similar situation exists in special relativity with our choice of contravariant or covariant vectors.
[6] This completeness
of the orthonormal basis is not the same as the mathematician’s notion of the completeness
of Hilbert space, which means that every Cauchy sequence in the space converges to
a point in the space according to the metric defined by the norm.
[7] See note 4; then take the case where |α> and |β> are eigenvectors: |ψ⟩ = a|φ1⟩ + b|φ2⟩. The probability of being found in eigenstate φ1 is |a|2, and the probability of being found in eigenstate φ2 is |b|2. Each of these eigenstates has a corresponding eigenvalue (a real number) which is the measured value of the physically observable dynamical property.
[8] Dirac himself avoided such contradiction by saying of his principle of superposition: Such further description should be regarded, not as an attempt to answer questions outside the domain of science, but as an aid to the formulation of rules for expressing concisely the results of large numbers of experiments.
(The Principles of Quantum Mechanics, 1930, ch. 1, sec. 2) He therefore follows Bohr in a purely instrumentalist notion of quantum theorizing, but at the expense of realism in theoretical physics.
[9] Apart from the mathematical reason given, a primary insight of quantum mechanics is that certain properties are only manifested as discretely quantized values. Elevating superpositions to the same level of reality as eigenstates would effectively de-quantize quantum mechanics.
[10] C. Monroe, D.M. Meekhof, B.E. King, D.J. Wineland, A ‘Schrödinger cat’ superposition state of an atom,
Science, 272 (1996), 1131-1136.
[11] By classical probability,
I only mean the basic probability theory that is conventionally taught in mathematics courses and can be applied to classical mechanics. I am not referring to the classical
interpretation of probability by Laplace, Pascal, et al.
[12] Refer to previous discussion of the wavefunction’s completeness relation. It is vain to argue that the fundamental particles are structureless,
for such a characterization only has meaning with respect to the known fundamental forces. At one time the nucleus was considered structureless; after the neutron was discovered, a strong
force mediated by gluons had to be introduced, under which even the nucleons are not structureless. We cannot be certain, then, that there is not some deeper force behind today’s fundamental
phenomena. Such deeper forces, if they exist, must be non-local, per Bell’s inequality.
[13] Aquino and Behmani (2007) have demonstrated that it is mathematically possible to reconstruct the initial spin state of an ensemble of particles by coupling it to another system for a sufficiently long period of time. This reconstruction of the density matrix allows us to say how many particles were in various x, y and z-spin states at the same time, but it does not circumvent the impossibility of determining an x-spin state and a z-spin state at the same time for a specific particle. G. Aquino and B. Mehmani, Simultaneous Measurement of Non-Commuting Observables
in: Beyond the Quantum (Leiden, Netherlands: World Scientific, 2007)
[14] Experiment conducted by Edward Fry and Randall Thompson in 1976. The researchers also demonstrated that the z-spin measurements of particle pairs were inversely correlated only probabilistically when taken along different axes, in proportion to the square of the angle between the two axes. This agrees exactly with the mathematical prediction of quantum mechanics, as the x component of spin is uncorrelated with the z component. Note that, mathematically, either particle might be considered to be in a superposition
with respect to the other particle’s state, but physically we only observe determinate eigenstates along a determinate axis with each measurement.
[15] The matrix and integral formalisms, also known as the Heisenberg and Schrödinger pictures, are formally equivalent in the sense of producing the same expectation values for all observables. A proof is in Appendix A of the cited paper. However, there are scenarios where the Schrödinger picture yields non-gauge invariant terms. This is due to an inconsistency in the Schrödinger picture arising from its supposition that there is always a lower bound to the free field energy. When this lower bound is removed, the Schrödinger picture becomes gauge invariant like the Heisenberg picture. [Dan Solomon, The Heisenberg versus the Schrödinger picture and the problem of gauge invariance,
Apeiron (2009), Vol. 16, No. 3., pp. 376-407.]
[16] Direct measurements of position are no more accurate than a fraction of an angstrom (10-10 m), and the same is true for momentum, if it is measured by successive position readings. This only takes us to 10-20, nowhere near ħ/2 = (h/2π)/2 = h/4π = 5.273 x 10-35. However, indirect measures may take us to the Heisenberg limit. An electron’s energy can be measured to within 0.01 eV, or 1.602 x 10-22 joule, which corresponds to a momentum (√2mE) of 1.7 x 10-26 kg-m/sec. If such an electron could simultaneously be confined to within an angstrom, we might test the Heisenberg limit. Recently, Higgins et al. (2007) have made the first Heisenberg-limited measurement of optical phases using a polarization interferometer. [B.L. Higgins, D.W. Berry, S.D. Bartlett, H.M. Wiseman, G.J. Pryde, Entanglement-free Heisenberg-limited phase estimation,
Nature (2007), Vol. 450, pp. 393-396.]
[17] See C. Cohen-Tannoudji et al., Quantum Mechanics, pp. 187-188. Any two operators Q, P with the commutation relation [Q, P] = iħ will have a continuum of eigenstates with eigenvalues for every real number. Heisenberg’s uncertainty principle is a consequence of this commutation relation, so it is senseless to invoke this principle as a basis for denying the reality of infinitesimally varying position states. On the contrary, such continuity is explicitly presupposed. If space were grainy
on the Heisenberg scale or any other scale, the canonical commutation relation of position and momentum could not hold.
[18] This is clearer in the Heisenberg picture, which shows explicit time dependence in the commutation relations.
[19] P.A.M. Dirac, The Principles of Quantum Mechanics (1930), ch. 4, sec. 24. Dirac interprets Heisenberg’s principle to imply that definite position implies infinite uncertainty in momentum and therefore unacceptably infinite possible energy. He concludes that position eigenstates and momentum eigenstates cannot be attained in practice,
though the force of his argument is grounded in an a priori claim that definite position implies potentially infinite energy. The impossibility of a position eigenstate is contradicted by his earlier statement that any result of a measurement of an observable must be one of its eigenvalues.
(ch. 2, sec. 12.) Note that we are talking about measurements on a formal theoretical level, so the finite precision of our measuring instruments is not relevant here.
[20] This error of confining causality to spatiotemporal links between physical events has allowed physicists such as Hawking to make the metaphysically naïve claim that the universe is causally self-contained and needs no prior cause.
[21] This is not to say that the similarity between Schrödinger’s equation and the electromagnetic wave equation is purely accidental. It could be the case that the latter is a specific application of the former. Nonetheless, the quantum wavefunction and classical wave are conceptually distinct; one exists in Hilbert space, the other in real space.
[22] Dirac, op. cit., ch. 1, sec. 3.
[23] The materialists, much like modern physicists, were given to making grandiose philosophical claims supposedly proven by physical science. Among these was the claim that the law of conservation of energy disproved theism. Then, as now, scientists suffered from a narrow metaphysical horizon, supposing that their particular notion of causality was the only possible kind.
© 2000, 2006, 2011 Daniel J. Castellano. All rights reserved. http://www.arcaneknowledge.org
| Home | Top |